publications

2024

1. 

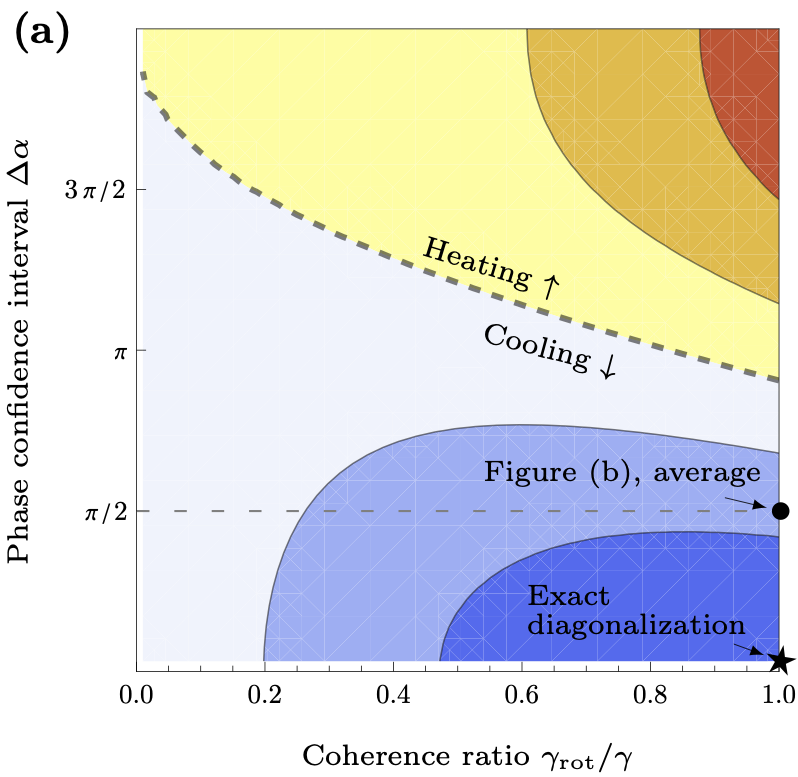   Cooling Limits of Coherent Refrigerators

   Rodolfo R. Soldati, Durga B. R. Dasari, Jörg Wrachtrup, and Eric Lutz

   Oct 2024

   Abs DOI arXiv

   Refrigeration limits are of fundamental and practical importance. We here show that quantum systems can be cooled below existing incoherent cooling bounds by employing coherent virtual qubits, even if the amount of coherence is incompletely known. Virtual subsystems, that do not necessarily correspond to a natural eigensubspace of a system, are a key conceptual tool in quantum information science and quantum thermodynamics. We derive universal coherent cooling limits and introduce specific protocols to reach them. As an illustration, we propose a generalized algorithmic cooling protocol that outperforms its current incoherent counterpart. Our results provide a general framework to investigate the performance of coherent refrigeration processes.

2023

1. [Thesis] Quantum Cooling: Thermodynamics and Information

   Rodolfo R. Soldati

   Oct 2023

   Abs DOI

   The theory of cooling is an important corner of thermodynamics, underlying many modern technological applications. As the field of quantum thermodynamics advances, refrigeration techniques must keep pace to fuel the innovations of quantum technologies. We study quantum cooling from its foundations to laboratory implementations within the specific paradigm of heat bath algorithmic cooling. Our study includes a detail modeling of experimental imperfections and establishes the fundamental cooling limits of the model, consolidating the algorithm as a viable quantum refrigeration method. Next, by developing the notion of virtual qubits, we demonstrate a cooling-boost protocol fueled by quantum coherences which is robust to experimental implementations. Aiming at aiding in the progress of refrigeration technologies, we conclude by studying the zero temperature equilibrium properties of a many-body system that can accommodate an autonomous quantum absorption refrigerator, and calculate its entanglement and critical properties, two features that, like quantum coherences, promise to improve the performance of quantum coolers.

2022

1. 

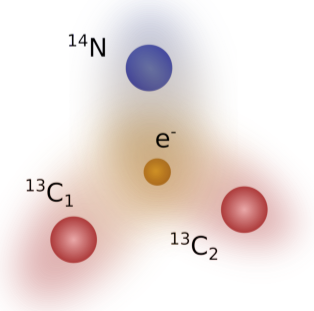   Thermodynamics of a Minimal Algorithmic Cooling Refrigerator

   Rodolfo Soldati, Durga Bhaktavatsala Rao Dasari, Jörg Wrachtrup, and Eric Lutz

   Phys. Rev. Lett., Jul 2022

   Abs DOI arXiv

   We investigate, theoretically and experimentally, the thermodynamic performance of a minimal three-qubit heat-bath algorithmic cooling refrigerator. We analytically compute the coefficient of performance, the cooling power and the polarization of the target qubit for an arbitrary number of cycles, taking realistic experimental imperfections into account. We determine their fundamental upper bounds in the ideal reversible limit and show that these values may be experimentally approached using a system of three qubits in a nitrogen-vacancy center in diamond.

2021

1. 

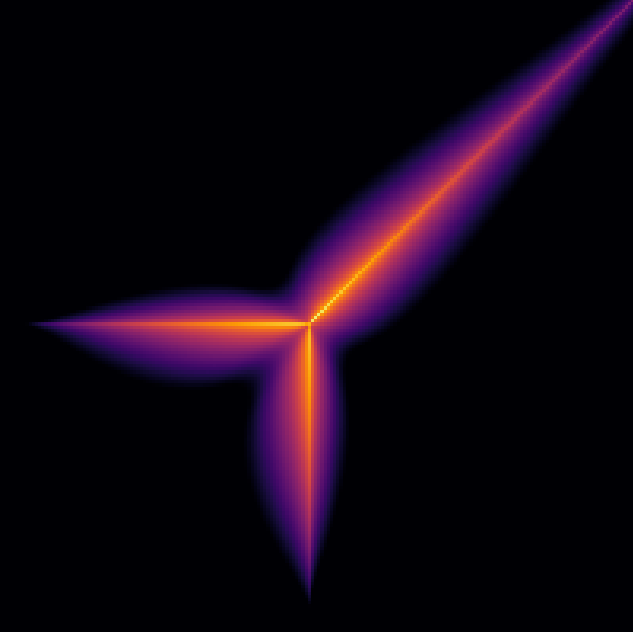   Multipartite Quantum Correlations in a Two-Mode Dicke Model

   Rodolfo Soldati, Mark T. Mitchison, and Gabriel T. Landi

   Phys. Rev. A, Nov 2021

   Abs DOI arXiv

   We analyze multipartite correlations in a generalized Dicke model involving two optical modes interacting with an ensemble of two-level atoms. In particular, we examine correlations beyond the standard bipartite entanglement and derive exact results in the thermodynamic limit. The model presents two superradiant phases involving the spontaneous breaking of either a \mathbbZ_2 or \mathrmU(1) symmetry. The latter is characterized by the emergence of a Goldstone excitation, found to significantly affect the correlation profiles. Focusing on the correlations between macroscopic subsystems, we analyze both the mutual information as well as the entanglement of formation for all possible bipartitions among the optical and matter degrees of freedom. It is found that while each mode entangles with the atoms, the bipartite entanglement between the modes is zero, and they share only classical correlations and quantum discord. We also study the monogamy of multipartite entanglement and show that there exists genuine tripartite entanglement, i.e. quantum correlations that the atoms share with the two modes but that are not shared with them individually, only in the vicinity of the critical lines. Our results elucidate the intricate correlation structures underlying superradiant phase transitions in multimode systems.

2. 

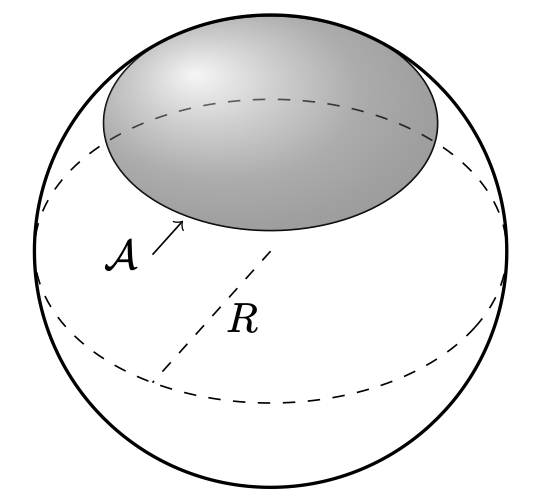   Universal Terms of the Entanglement Entropy in a Static Closed Universe

   Rodolfo Soldati, L. S. Menicucci, and N. Yokomizo

   Phys. Rev. D, Dec 2021

   Abs DOI arXiv

   Subdominant contributions to the entanglement entropy of quantum fields include logarithmic corrections to the area law characterized by universal coefficients that are independent of the ultraviolet regulator and capture detailed information on the geometry around the entangling surface. We determine two universal coefficients of the entanglement entropy for a massive scalar field in a static closed universe \mathbbR \times \mathbbS^3 perturbatively and verify the results numerically. The first coefficient describes a well known generic correction to the area law independent of the geometry of the entangling surface and background. The second coefficient describes a curvature-dependent universal term with a nontrivial dependence on the intrinsic and extrinsic geometries of the entangling surface and curvature of the background. The numerical calculations confirm the analytical results to a high accuracy. The first and second universal coefficients are determined numerically with a relative error with respect to the analytical values of the orders 10^-4 and 10^-2, respectively.

2016

1. 

   Poisson’s Spot and Gouy Phase

   I. G. da Paz, Rodolfo Soldati, L. A. Cabral, J. G. G. Oliveira Jr, and 1 more author

   Phys. Rev. A, Dec 2016

   Abs DOI arXiv

   Recently there have been experimental results on Poisson spot matter wave interferometry followed by theoretical models describing the relative importance of the wave and particle behaviors for the phenomenon. We propose an analytical theoretical model for the Poisson’s spot with matter waves based on Babinet principle in which we use the results for a free propagation and single slit diffraction. We take into account effects of loss of coherence and finite detection area using the propagator for a quantum particle interacting with an environment. We observe that the matter wave Gouy phase plays a role in the existence of the central peak and thus corroborates the predominantly wavelike character of the Poisson’s spot. Our model shows remarkable agreement with the experimental data for deuterium D_2 molecules.